Problem 15-8 Source:
Adapted from problem 70 of the circuit list
Electrical Circuits I, School of Engineering, UFRGS, Prof. Dr. Valner Brusamarello.
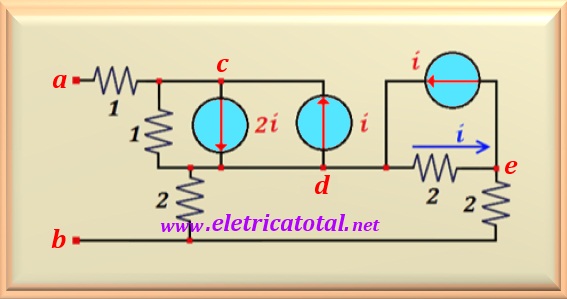
Determine the Thévenin equivalent for the circuit containing
only dependent sources in the circuit shown in Figure 15-08.1.
Solution of the Problem 15-8 -
Thévenin/Norton Method
As the problem statement says, the circuit has only dependent sources . So we already know that as there are no independent sources that can excite the circuit, Thévenin's Voltage will be
null . All that remains is to calculate the Thévenin resistance .
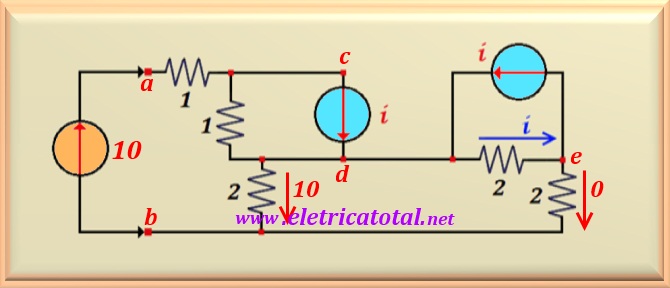
For this, we will excite the circuit with an external source of convenient value. We choose
a current source with a value equal to 10 A . It could be any other value.
By the circuit, we can already solve the parallel of the two current sources resulting in a single source of current of value equal to i .
See in the Figure 15-08.2 the modified circuit.
Let's make some considerations about the circuit shown in the figure above.
The current i signaled by the blue arrow (to the right
of the circuit)
will be confined between the source of current i and the resistor
of 2 ohms
(in parallel with the source), and as a result, in the resistor of 2 ohms
which is between the points e -b , no electric current will circulate.
This means that the potential of the point e is
the same potential of the point b. In this way, we can eliminate the resistor
of 2 ohms
which is between the b-e points. And so, the point e and the point
b can be united in a single point, because they have the same potential.
But there is no current circulating between these points as shown in the
Figure 15-08.3. As a consequence, we notice that the current of 10 A
that enters by the point a must necessarily pass through the resistance of
2 ohms that is connected between the points d - b . And this allows
we calculate the voltage between
these points, that is:
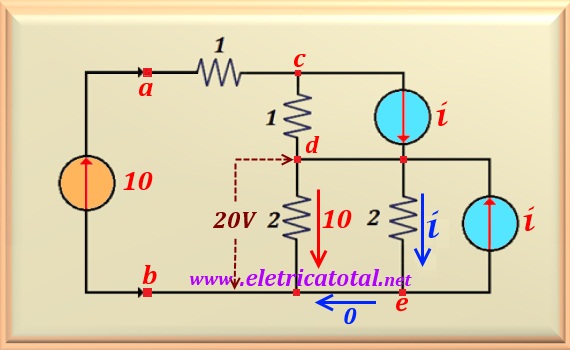
See the Figure 15-08.3 how the circuit was after these considerations.
Then, knowing the value of Vdb we easily calculate the value
of i.
As i = 10 A , this means that there will be no current through the
resistor of
1 ohm which is between the points c-d , because all the current
will be supplied by the source current i which is in parallel with the
resistor. In this way, we conclude that
Vcd = 0 volt. Then:
As we know the value of Vab and the current entering the terminal a,
we easily calculate the Thévenin resistance, that is:
Thus, we prove that a circuit containing only dependent sources, its Thévenin equivalent is represented only by Thévenin's resistance, because the Thévenin Voltage is zero.