Problem 13-11 Source:
Problem worked out by the website author.
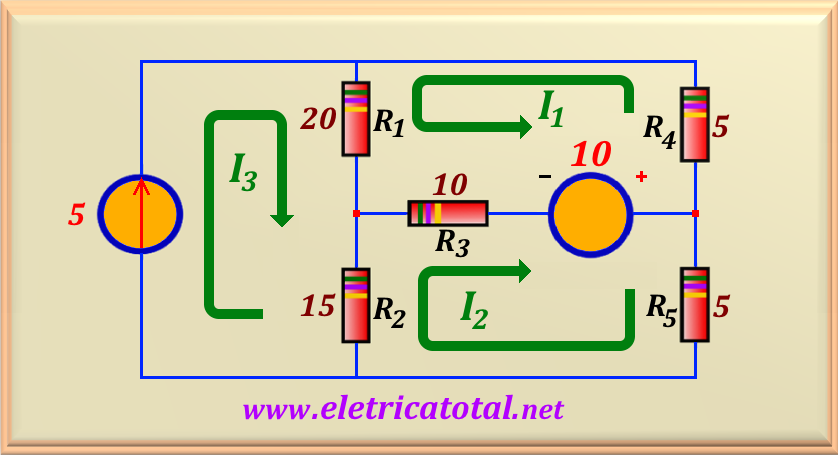
For the circuit shown in Figure 13-11.1,
using Kirchhoff's law, determine:
a) The currents in the circuit;
b) The power dissipated in R3.
Solution of the Problem 13-11 -
Kirchhoff's Method
Making use of the method of mesh voltages ( Kirchhoff's law ) we know that in a closed loop
the algebraic sum of the voltages must be equal to ZERO. Note carefully the meaning we take to the
currents in the circuit. It should be noted that the value of I3 is already known and is equal to 5 A .
Thus, we can write the following equations for the circuit:
And since we know the value of I3 it is not necessary to mesh I3. Then,
substituting the value of I3 in the above equations, we get the following system
from two equations to two unknowns, or
And now, solving by substitution or any other method, we find:
To find the value of the power dissipated in R3, we must calculate the current flowing
through that resistor. However, by Figure 13-11.1 we can easily see that
IR3 = I1 + I2. And so, calculating we have
IR3 = - 3.74 + 4.08 = 0.34 A. So, using
the power equation, we find