Problem 10-16    Source:   Problem elaborated by the author of the site.

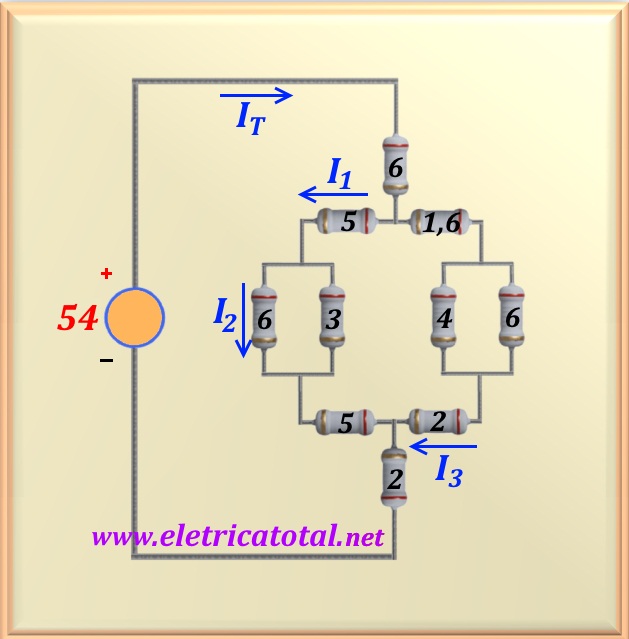
In the circuit show in Figure 10-16.1, determine:

a) The value of I_T.

b) The values of I₁ , I₂ and I₃.

c) The power dissipated in the resistor of 1.6 Ω.

Solution of the Problem 10-16

Item a

In order to calculate the current I_T, one must calculate the equivalent resistance of the entire circuit. It is possible to calculate the equivalent resistance of the left branch where the currents circulate I₁ and I₂. Making the parallel of the 6 ohms and 3 ohms resistors yields 2 ohms. Now there are three resistors in series. In addition to its values, R_esq = 5 + 2 + 5 = 12 ohms. Now doing the right branch, there is the parallel of the 6 ohms and 4 ohms resistors resulting in 2.4 ohms. Then, the equivalent resistance of the right branch is R_dir = 1.6 + 2.4 + 2 = 6 ohms

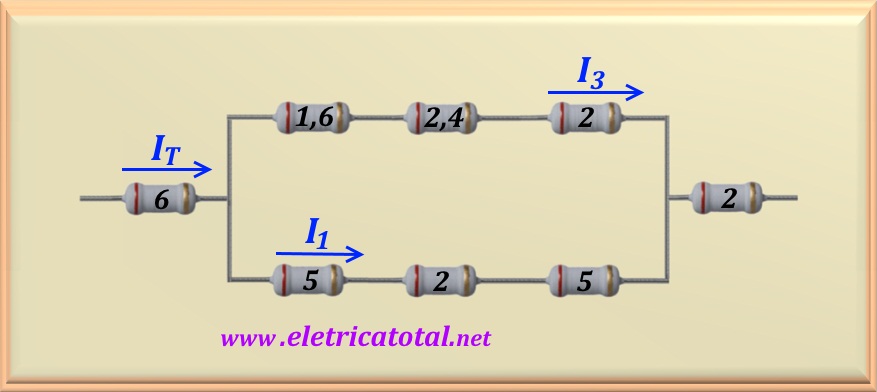
The Figure 10-16.1 shows how simplified the circuit was. The next step is to determine the value of the parallel between R_esq and R_dir, values as calculated above.

Using the equation that allows to calculate the parallel between two resistors:

Making the substitution by the numerical values and performing the calculation:

To find the equivalent resistance value of the whole circuit, simply calculate the series of three resistors. Like this:

To compute the I_T value, simply apply the Ohm law, or:

Item b

To calculate I₁ and I₃ simply apply a current divider to the circuit above. Remembering that R_esq = 12 Ω   and   R_dir = 6 Ω, so:

In order to find the value of I₂, we must pay attention to the initial circuit and verify that this current is a derivative of I₁. Then, by applying a current divider:

Item c

In order to calculate the power that the 1.6 Ω resistor dissipates, it is sufficient to verify that the current circulates through it is I₃ = 3 A. Then, applying the equation studied in Chapter 7 we have: