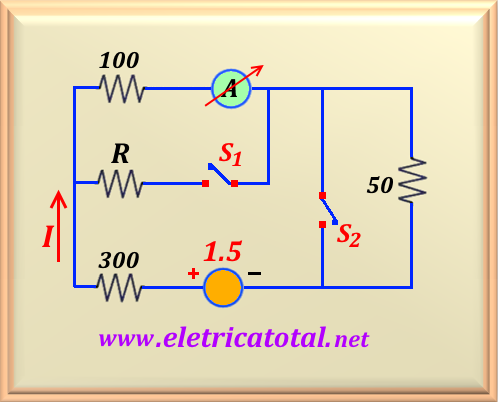
Problem 10-20    Source:   Problem F6 of the list of UFRGS - 2016.

The current measured by the ammeter A in the circuit of Figure 10-20.1 it is the same when both keys are open or closed. Determine the value of R.

Solution of the Problem 10-20

Case 1

Let's consider the case of the two open keys. In this situation, we easily calculate the current I that circulates through the ammeter, since the three resistors are in series. So, using Ohm's law, we have:

This is the ammeter A reading.

Case 2

Now let's consider the case where the two keys are closed. Note that in this case the resistor of 50 Ω is out of the circuit. However, in this case, we know that the ammeter reading must be the same as in case 1. Soon, A = 10/3 mA. Note that in this case, the current I is different from the reading of the ammeter A . In this way it is possible to calculate the voltage drop on the resistance of 100 Ω, that is:

So, without difficulties, we noticed that the voltage drop on the resistor of 300 Ω it's the same as V₃₀₀ = V - V₁₀₀ = 1.5 - (1/3 ) = 7/6 volt. Therefore, we can calculate the current I that flows through the resistor of 300 Ω. Then:

So the current flowing through the resistor R is the difference between the current I and the current measured by the ammeter A. Therefore:

Note that the voltage across resistor R is the same voltage across the resistor from 100 Ω, because the two are in parallel. So, we have V_R = V₁₀₀. And the value of R will be: