Problem + Hard 13-2    Source:   Problem worked out by the website author.

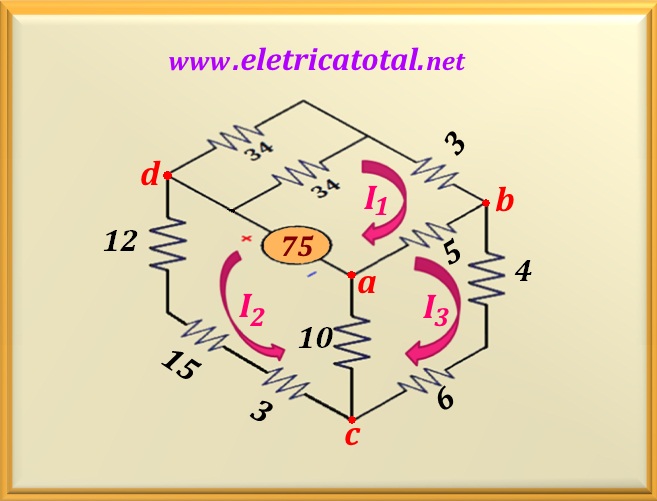
For the circuit shown in Figure 13-02.1, determine:

a) the values ​​of I₁, I₂ and I₃;

b) the value of the current flowing through the voltage source.

Solution of the Problem + Hard 13.2

Item a

At point d there are two resistors connected in parallel. Then you can replace them for an equivalent value equal to 17 Ω. Establishing mesh equations. Starting with I₁:

For the I₂ loop, we get:

And finally, for I₃:

So we have a system of three equations in three unknowns that can be solved by any method. Using Octave, you find the following values:

Item b

To find the current flowing through the voltage source, we must do the node equation for node a. Then:

Note that I₂ + I₃ is the current flowing between points c - a and I₁ - I₃ is the current flowing between points b - a. Soon, I₃ cancels and we get:

It should be noted that the voltage source delivers a power to the circuit equal to the product of the source voltage by the current passing through it. Then:

Note the negative value of the result, as the voltage source supplies power to the circuit. This power must be equal, in module, to the power dissipated by all the resistors that make up the circuit. We leave it as an exercise for the student to prove this affirmation.