Problem 11-12    Source:    Problem 37 and 60 - List of Electrical Circuit Exercises I - School of Engineering - UFRGS - 2013 - Prof. Dr. Valner Brusamarello.

In the circuit shown in Figure 11-12.1, it is known that the ammeter A measures 3 A. Determine the value of the current source I.

Solution of the Problem 11-12 -  Method of Transforming Sources

When solving this problem, it is important to remember that an ideal ammeter has zero internal resistance. Taking this into consideration, we realize that R₅ and R₆ can be eliminated from the circuit, as they are short-circuited by the ammeter. Furthermore, it appears that the current i that circulates through R₆ is nullified. As a consequence, the current source 5 i (on the left of the circuit) has its value equal to zero. And as we know, a zero value current source is an open circuit. Therefore, it can be removed from the circuit, as well as R₁ which is in series with this source. Note that the current source I₂ which is in series with R₆ is short-circuited and can be eliminated from the circuit. Therefore, we can simplify the circuit above as shown in Figure 11-12.2.

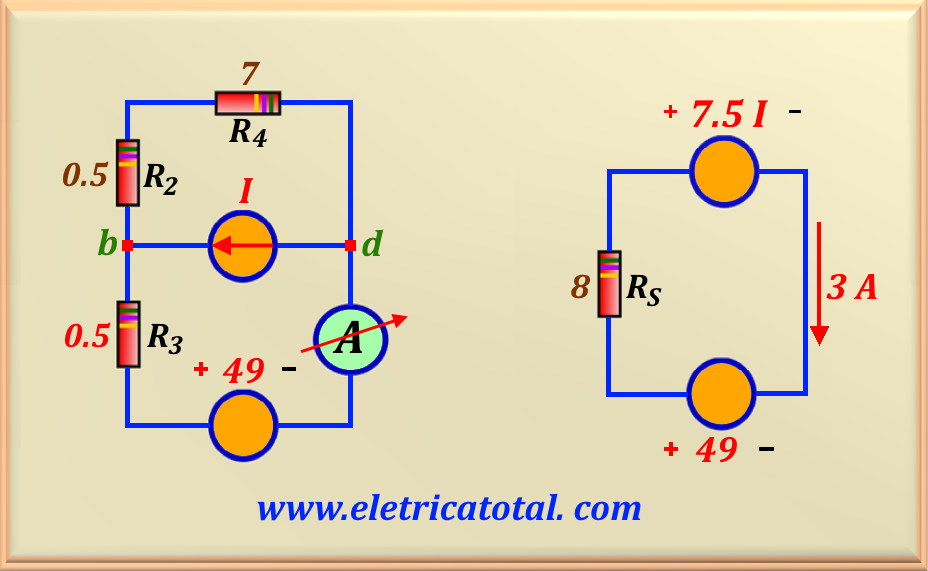
From the circuit we see that R₂ and R₄ are in series. Therefore, we can add their values ​​obtaining a resistor of 7.5 Ω. In this case, we have a current source in parallel with a resistor. Then it is possible to use source transformation and obtain a voltage source of 7.5 I in series with a resistor of 7.5 Ω. And this 7.5 Ω resistor is in series with the resistor R₃ = 0.5 Ω. So, adding their values ​​we obtain a resistor of 8 Ω which we call R_S, as can be seen in the circuit on the right in Figure 11-12.2. Note that a series circuit resulted and, from the information given by the ammeter, we know that a current of 3 A circulates in this circuit. Therefore, using Kirchhoff we will determine the value of I, or:

Solving this equation we find the value of I.