Problem 2.12
Source: Problem elaborated by the author of the site.
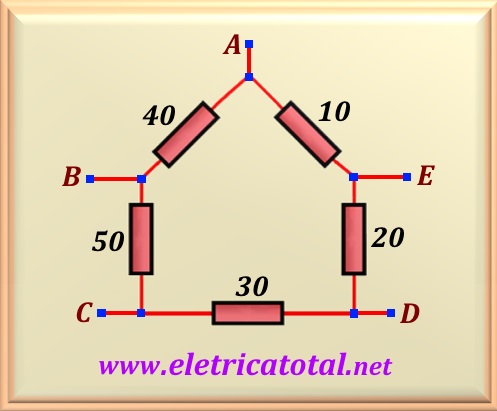
In the circuit shown in the Figure 02-12.1, find the equivalent resistance between the following terminals:
a) A-D.
b) C-E.
a) B-D when the C-E terminals are short-circuited.
Solution of the Problem 2.12
Note that we are calculating the equivalent resistance between the A-D
terminals. Thus, the resistors that lie between the AED points (series
resistors) total 30 ohms, while the resistors that lie between the ABCD points ( resistors in series) total an equivalent value of
120 ohms. Now, from the circuit, it is easily concluded that these two
groups of resistors are in parallel. So, to solve this item just calculate the parallel between 30 ohms and 120 ohms. Soon:
Similarly to the previous item, we must calculate the equivalent resistance value
between the points C-D-E. As the resistors in this branch are in series,
just add their values resulting 30 + 20 = 50 ohms. And in the C-B-A-E
branch, we have 50 + 40 + 10 = 100 ohms. Thus, as these two sets of
resistors are in parallel, we obtain:
To solve item c, we must realize that when shorting the CE
terminals, the resistors between CD and ED points stayed in
parallel. Therefore, the equivalent resistance between the points C-E
and D totals:
Now we must pay attention to the C-B-A-E branch. Note that the resistor
between CB points, when shorting CE points, will be parallel to the equivalent resistance between
BAE points. Then the equivalent resistance between the points B-CE will be:
And obviously these two sets of calculated resistors are in series with each other.
Thus, the equivalent resistance value between the B-D points is: