Problema + Difícil 10-1 Source:
Problem elaborated by the author of the site.
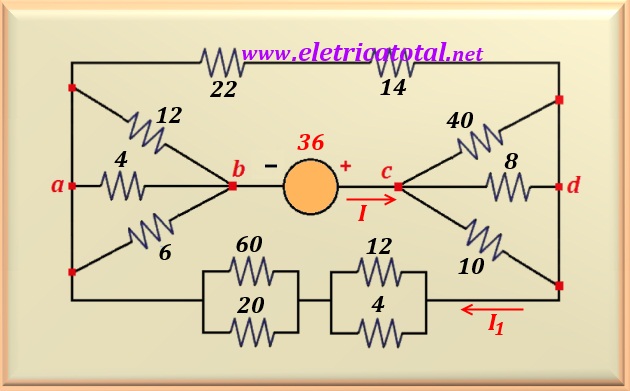
Determine the value of I1 and I in the circuit shown in
Figure 10-01.1.
Solution of the Problem + Hard 10-1
Note that the three resistors that are
between the nodes a-b, are in parallel. The same thing happens with the three
resistors that are between the nodes c-d. Calculating the parallel of these resistors,
results in an equivalent resistance of 2 ohms for those between the nodes
a-b and 4 ohms for those that
are between the c-d nodes. In the upper part of the circuit, interconnecting the nodes a-d,
there are two resistors in series. Carrying out the calculation, it results in a resistance
equivalent of 22 + 14 = 36 ohms. At the bottom of the circuit,
also interconnecting the nodes a-d, there are two sets
of resistors that are in parallel. Solving this parallel, results in 15 ohms
for the left set and 3 ohms for the right set.
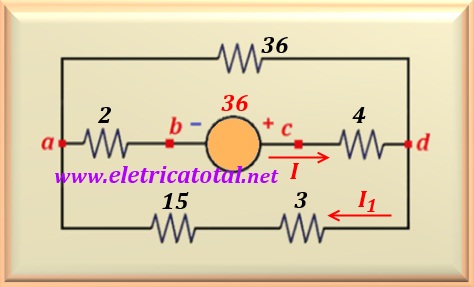
In Figure 10-01.2, the reduced circuit is shown after the calculations of the
several associations.
Note that the current I1 continues to flow through the resistors in the part
bottom of the circuit.
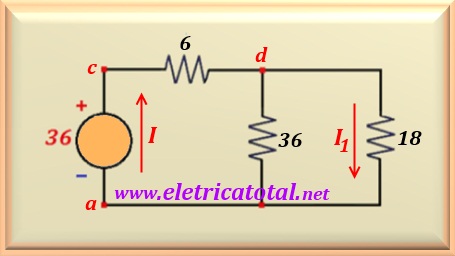
In Figure 10-01.3, the topology of the circuit has been changed a little,
evidencing that the
6 ohm resistor that is between the c-d nodes, is a result of the
Series association of the two resistors that were in series with the voltage source.
The resistors that connected the nodes a-d were placed in parallel.
And through the 18 ohms resistor, I1 circulates.
To calculate the value of I, you must first calculate the parallel
18 and 36 ohm resistors. Carrying out the calculation, the result is the value
of 12 ohms. Adding to the 6 ohms resistor (since they are in series)
find the equivalent resistance of the entire circuit, that is:
From this moment on, the value of I can be calculated. Like this:
To calculate I1, a current divider is applied.
Substituting I for its numerical value, we find